In a recent paper, we presented a new computational method for molecular dynamics which uses the Backward-Euler scheme to solve the classical Langevin dynamics equations. Parameters for the simulation include a target temperature T, a time step t, and a cutoff frequency 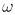_c. We showed for a harmonic oscillator system that the cutoff frequency can be set as 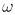_c=kT/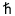 in order to mimic quantum-mechanical behavior. We now continue this investigation for a nonlinear case: a diatomic molecule governed by a Morse bond potential. Since approximate quantum-mechanical energy levels are explicitly known for this model, a comparison of energies can be made with molecular dynamics results. By performing dynamics runs for a wide range of temperatures and calculating mean energies, we find a very good agreement between these energies and quantum mechanical predictions. Vibrational excitation begins at temperatures around 800 K, and for higher temperatures both energy curves (molecular dynamics and quantum mechanics) approach the classical prediction of 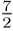kT energy per molecule. Future investigations will focus on more general nonlinear potential functions employed in force fields of nucleic acids and proteins

Click to go back to the publication list

Webpage design by Igor Zilberman